HARESH LALVANI 2007-2008

QUANTUM TOPOLOGY

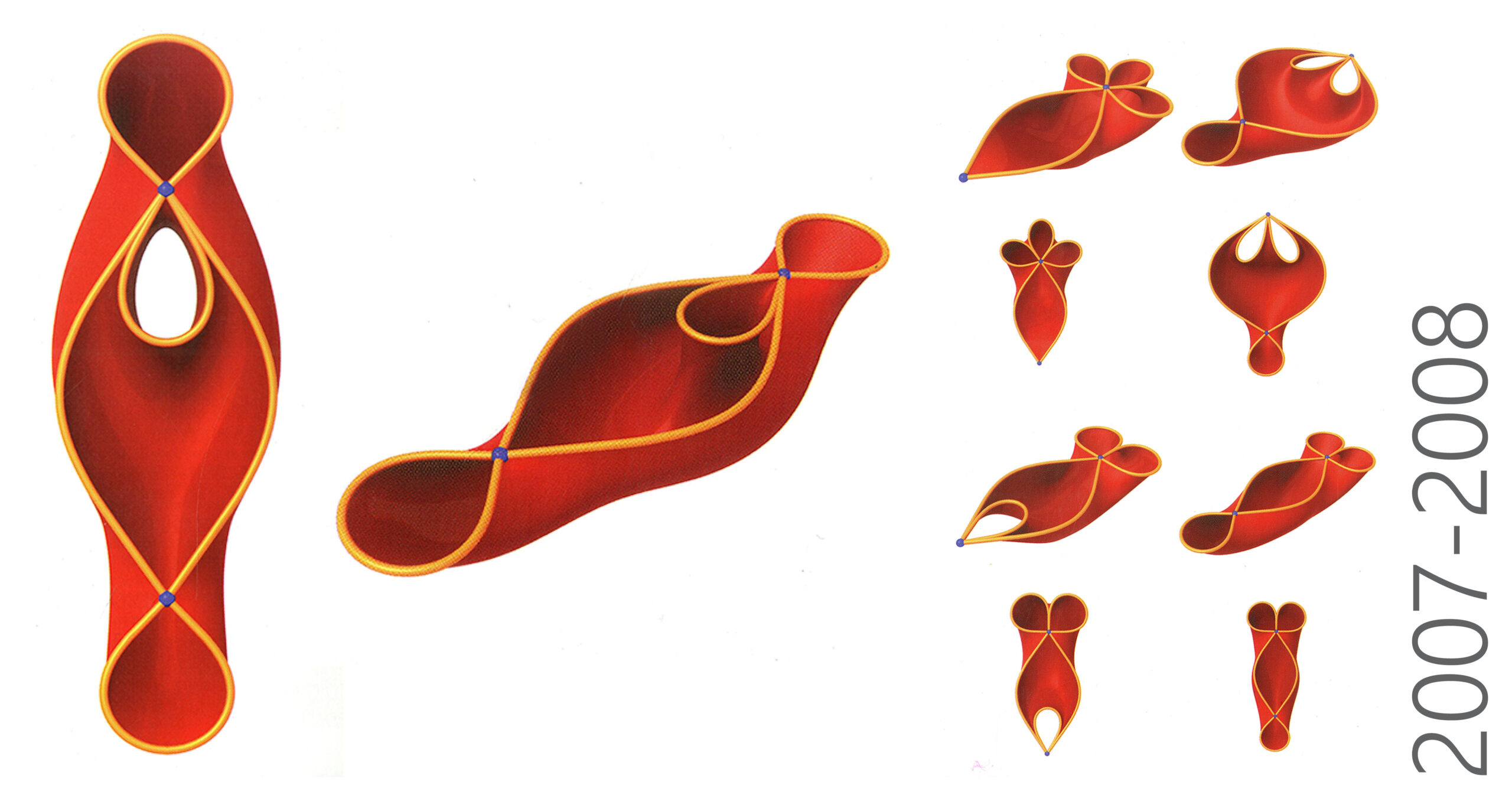
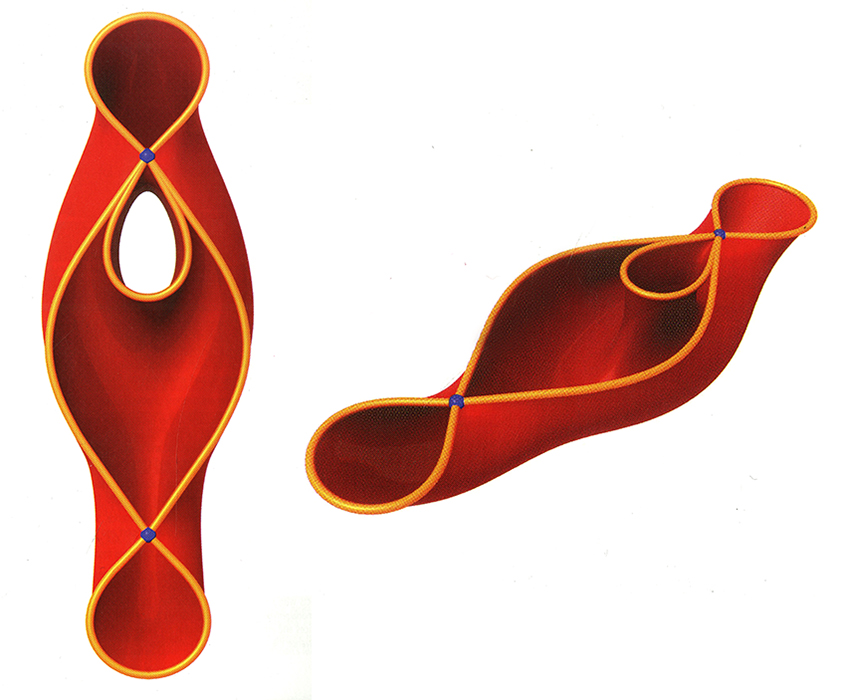
A genomic approach to topology leads to its quantization. This type of quantum topology generates new topological structures some of which are displayed here from ongoing work.  Our concept of morphological continuum leads to dynamic states of these that morph from one to another, resulting in a paradox: discrete (changes in topology) emerges from continuous (changes in geometry).

The surfaces shown here have a singularity defined by a single emanating vertex in a manner reminiscent of big-bang and black-hole morphologies in physics. These surfaces have non-Eulerian topologies in that they violate the Euler characteristic c (Greek character ‘chi’, and named after the great Swiss mathematician Leonhard Euler) that defines different topologies. For the simplest topologies, namely, polygons, c = 1-g, where g is the number of holes (genus).  The eight examples shown (each in two views) are all pentagons with varying number of vertices and holes, each defining a single continuous space bound by a single smooth membrane, and having varying Euler characteristic (1 in the equation is replaced by 0, -1, -2, -3, …….). This idea extends to higher-dimensional structures.

The natural way to physically construct these is using fluids or gravity acting on morphable membranes. Generative quantum topologies lead to new ways of shaping architectural space, and their inter-morphings lead to new adaptable architectural topologies.

(computer modeling: John Gulliford and Peter Van Hage)