HARESH LALVANI 2012-2013

EMERGENT TOPOLOGY

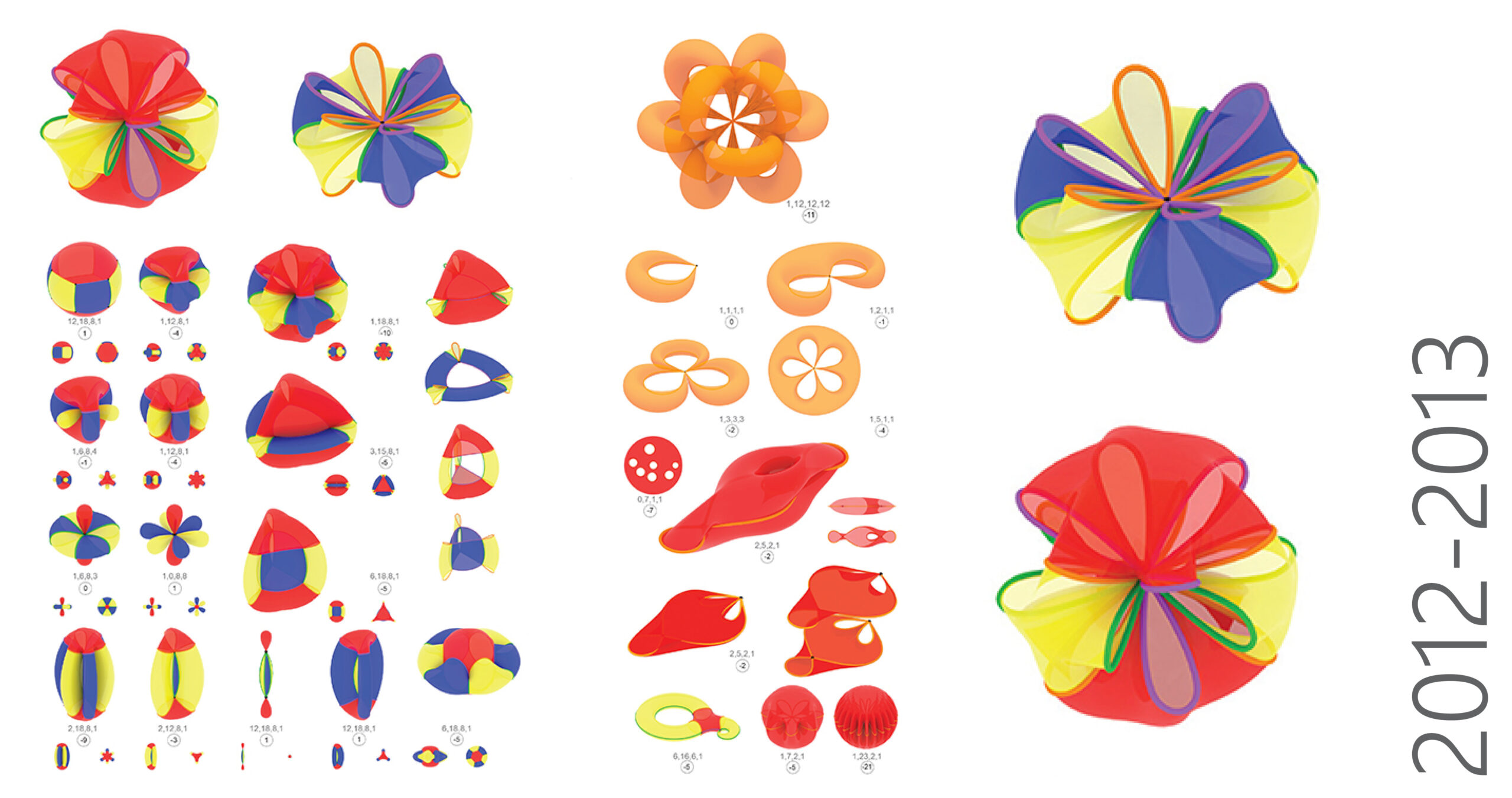
In the biology of form, every shape is a fleeting instant in a continuum of changing shapes. The morphological genome captures these changes systematically and exhaustively. Snapshots of single structures from this continuum are shown by activating Gene1 and Gene3 of the morph genome. The continuous morphing between the single structures leads to “emergence” of new topologies.

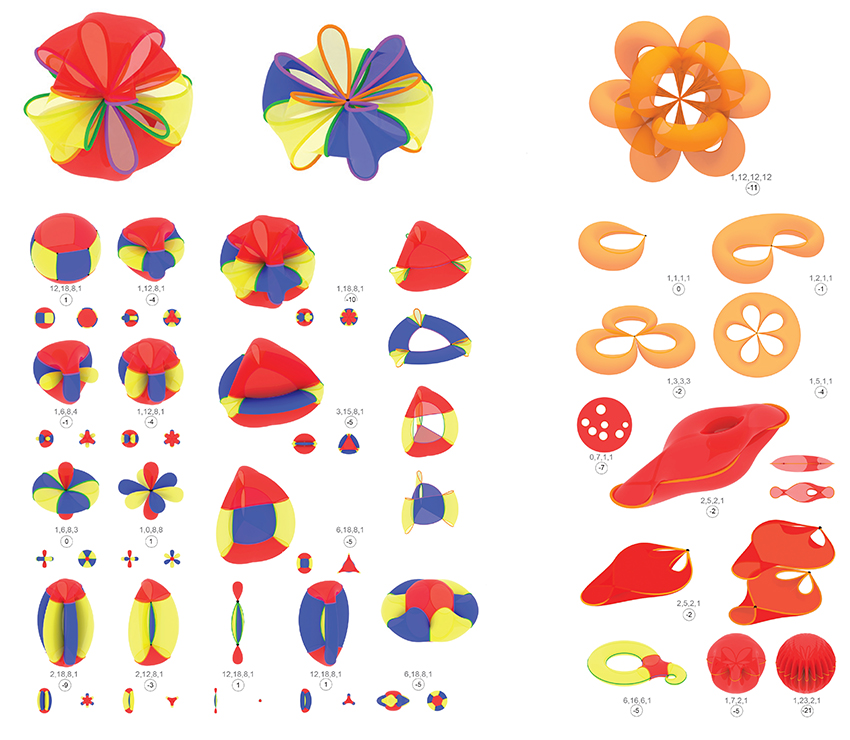
Gene1 generates topological structures with a single color (shapes in red, this page). These structures, mapped in an infinite 4-dimensional space, are indexed by four numbers corresponding to V, E, F, C : the number of Vertices (points), Edges (lines), Faces (planes or surfaces), and Cells (”volumes”) in any 3-dimensional structure. These four numbers are given under each structure along with the number inside which equals V-E+F-C. Interesting cases are when a group of vertices collapse to a single point and, in doing so, morphing the shapes of the cell, faces and edges, but keeping their numbers unchanged. These structures violate Euler’s celebrated topological equation V-E+F-C=1-g, where g is the genus of the structure. New topological elements “emerge” from the continuous transformation process.

Activating Gene3 of the morphological genome leads to “expression” of additional colors exhibiting derivative topologies. Blue, yellow, orange, green and violet are added to red. The shapes in red, yellow and blue shown here (left page) are examples from one family of a continually collapsing spherical polyhedron (top left) whose points have gradually joined in various ways while edges too have gradually vanished in the process. Shapes in orange (top images, this page) are another interesting group of topologies as they comprise polygons folding over themselves to contain space.

Physical construction of continually morphing shapes pose interesting challenges. Using pliable or stretchable materials is the obvious choice. More interesting cases are when rigid materials lead to pliable geometries that are digitally controlled. These usually are “lo-res” faceted versions of smooth surfaces. At the nano-scale, building bottom-up will require a morph-code to be embedded in matter.

Continually morphing topologies force us to think of new physical solutions so structures can change responsively to meet the complex needs of designs that adapt and evolve with time. The morphological genome provides a formal starting point.

Computer Modeling/Rendering: Patrick Donbeck (Lalvani Studio)