Haresh Lalvani 2021-2022
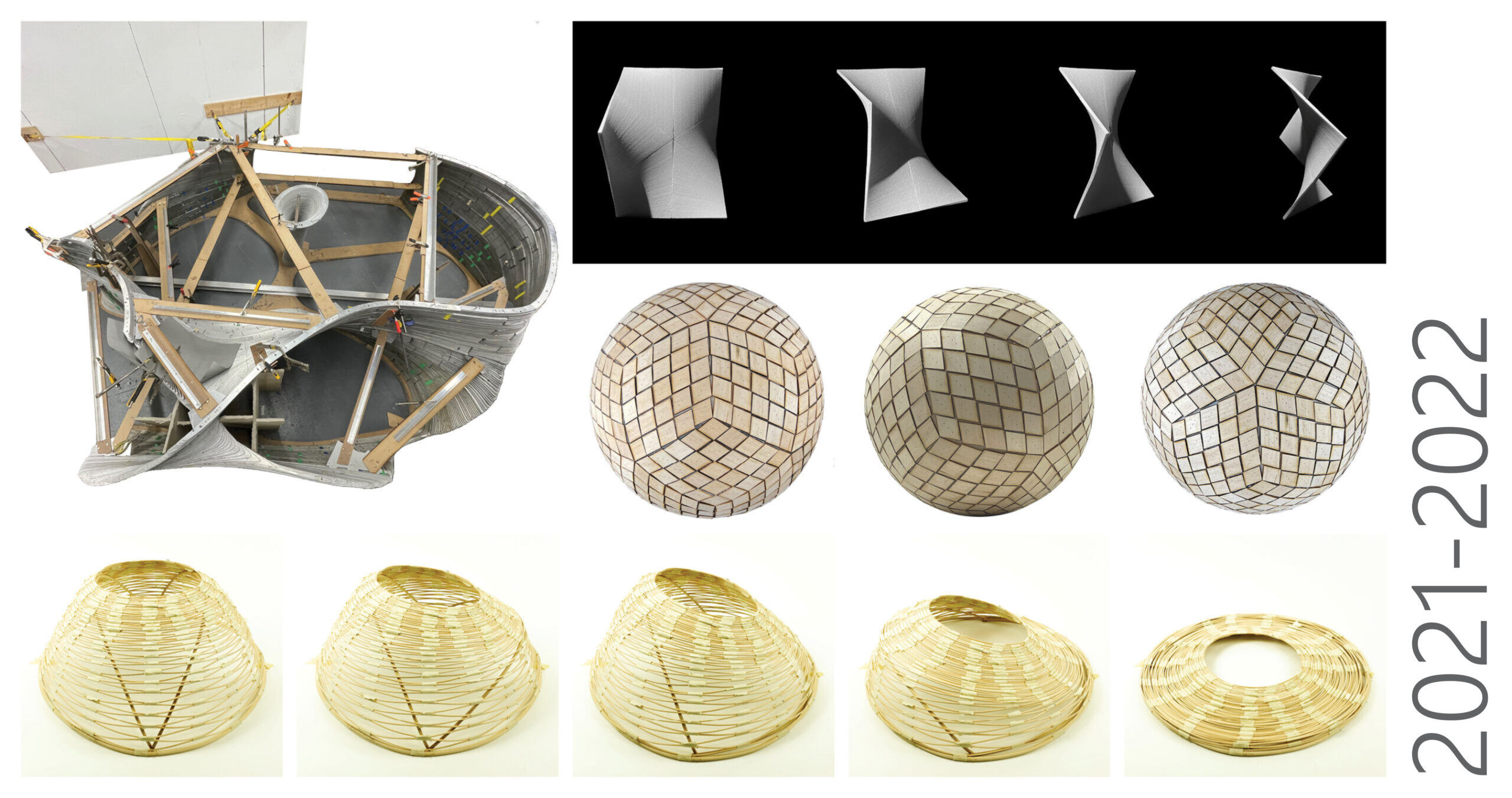
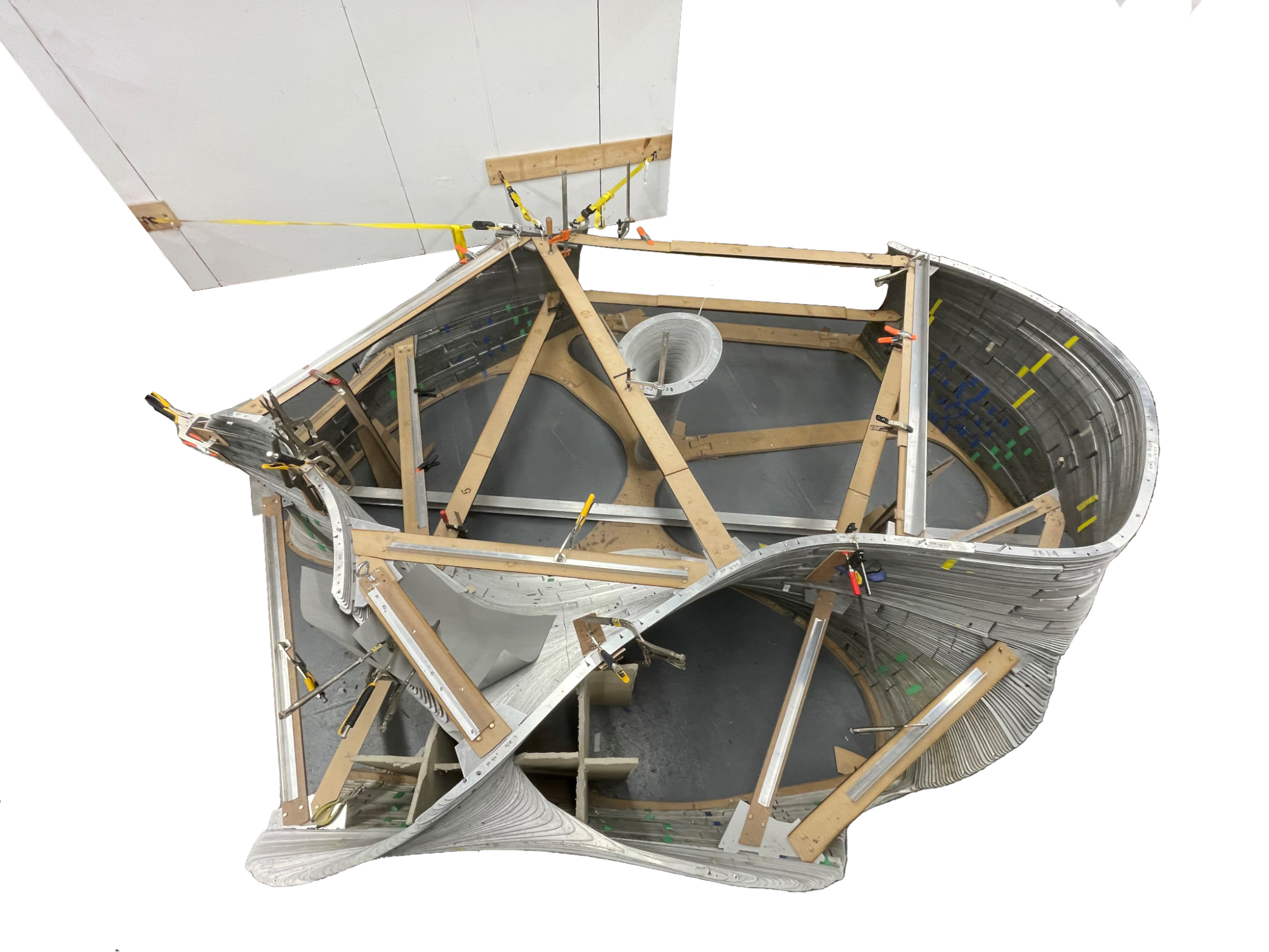
3D HYPER SURFACE: Phase 3
In this continuing project of constructing a 3D one-sided minimal surface comprising six “layers” (Layers 1-6) of laminated contoured alucobond pieces, space relocation required disassembly and reassembly of Layers 3 and 4 and led to improvements in achiev- ing dimensional accuracy of assembly. New construction features (cross-bars, internal rods) were introduced. Free-standing walls required tension attachments to the outer walls. Layers 1 and 2 are ready for assembly. This will be followed by development of the foundation plate and on-site installation sequence.


[Credits:]
Principal Investigator: Haresh Lalvani
Research Interns: Matthew Mitchell, Quinten Oxender
UG Research Assistants: Tron Le, Dillon Marlow
Consultant: Mohamad Fahan
Industrial Support: Milgo-Bufkin
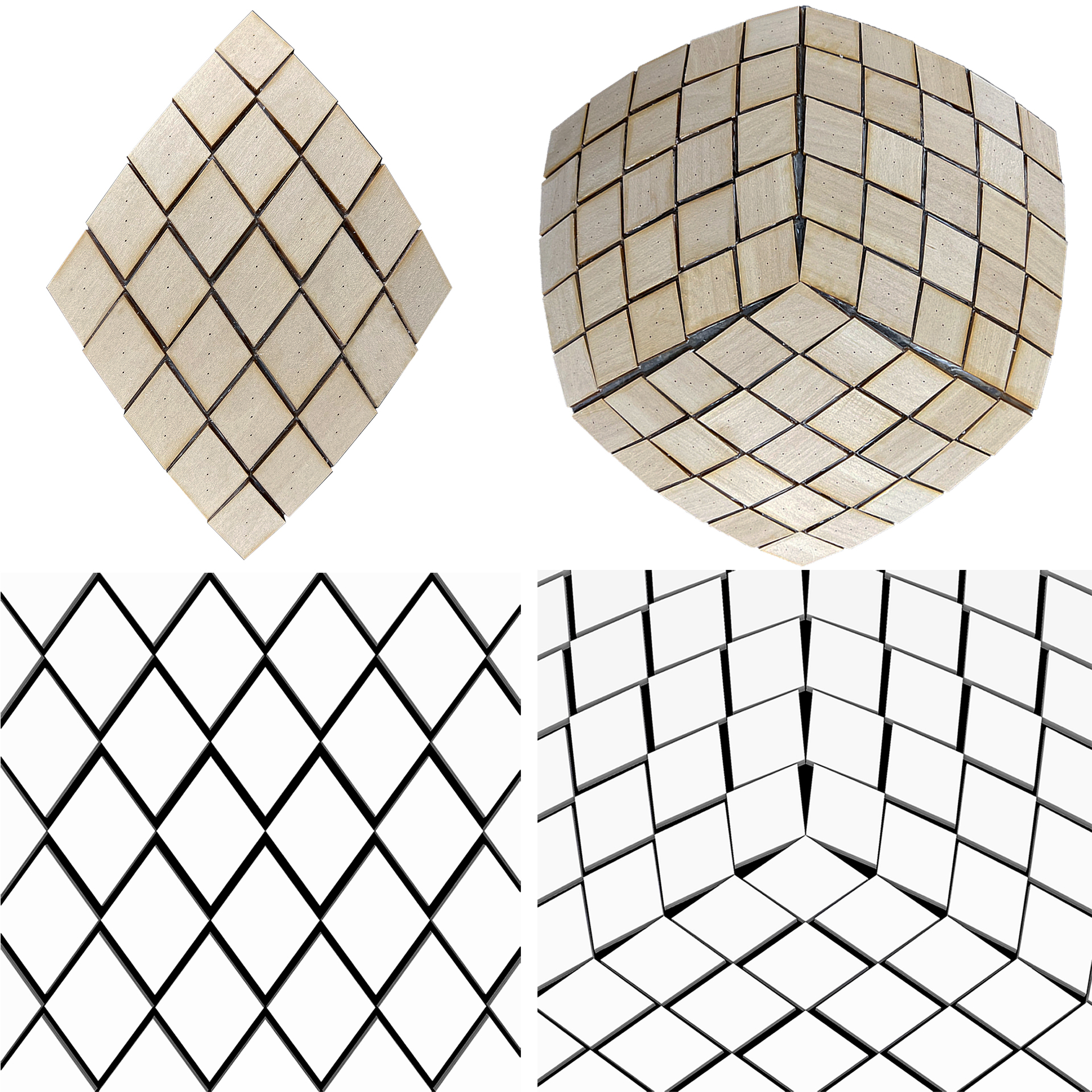
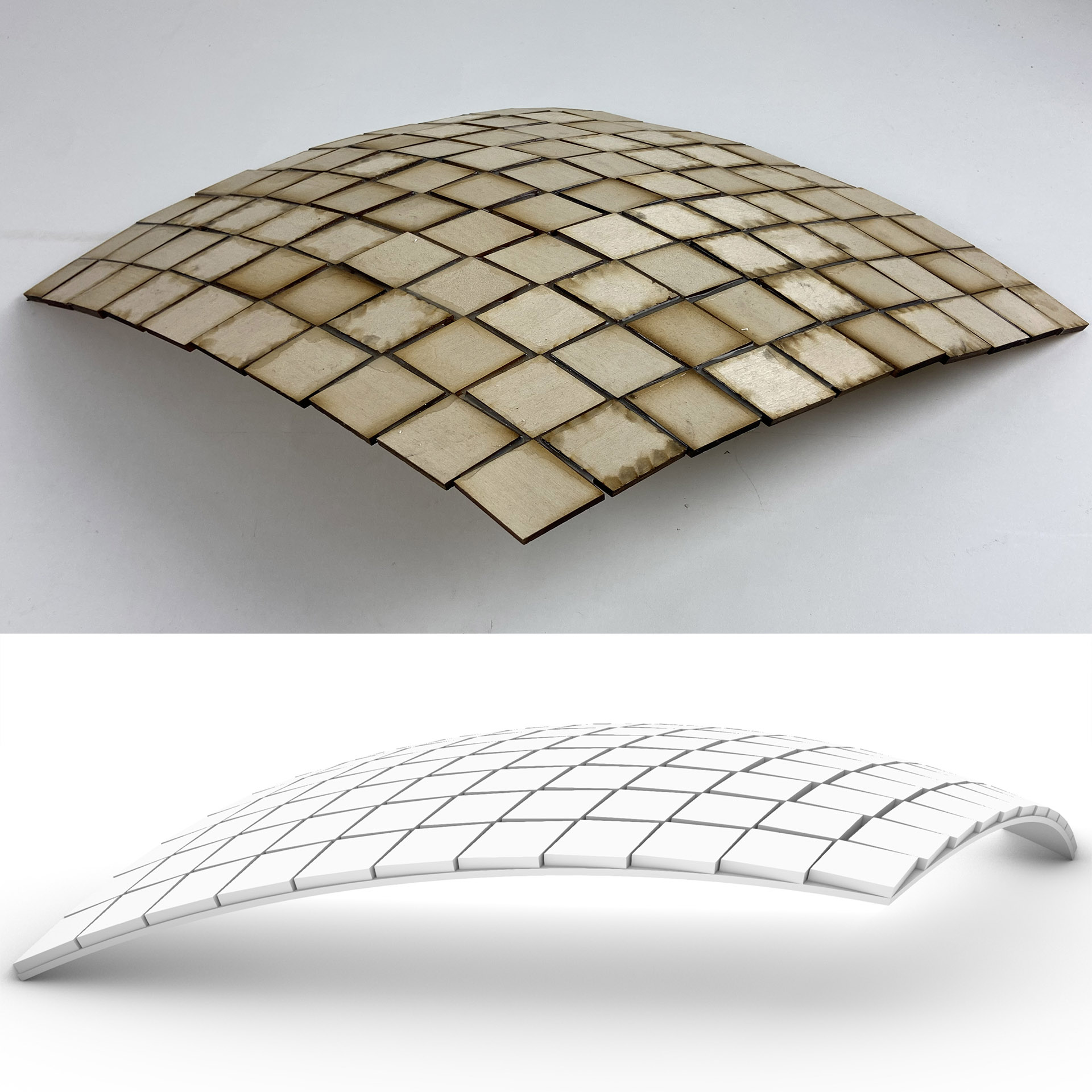
CURVED PENTILE SURFACES
A new type of brick (tile) construction requiring variable gaps provides a way to build compound curved surfaces with positive and negative curvature using identical flat non-standard bricks (tiles). Spheres using a 72-degree rhombus from our Pentiles set (n=5 case) are our starter examples (spheres, cylinders, saddles) for more complex space- filling curved surfaces. Variable gaps bypass the mathematical limitation of 60 (or 120) identical tiles with no gaps permitted to cover a sphere. Works of Guastavino and the structure of viruses have been inspirational.



[Credits:]
Principal Investigator: Haresh Lalvani
UG Research Assistants: Tron Le, Dillon Marlow, Rithika Vedapuri, Emma Xu
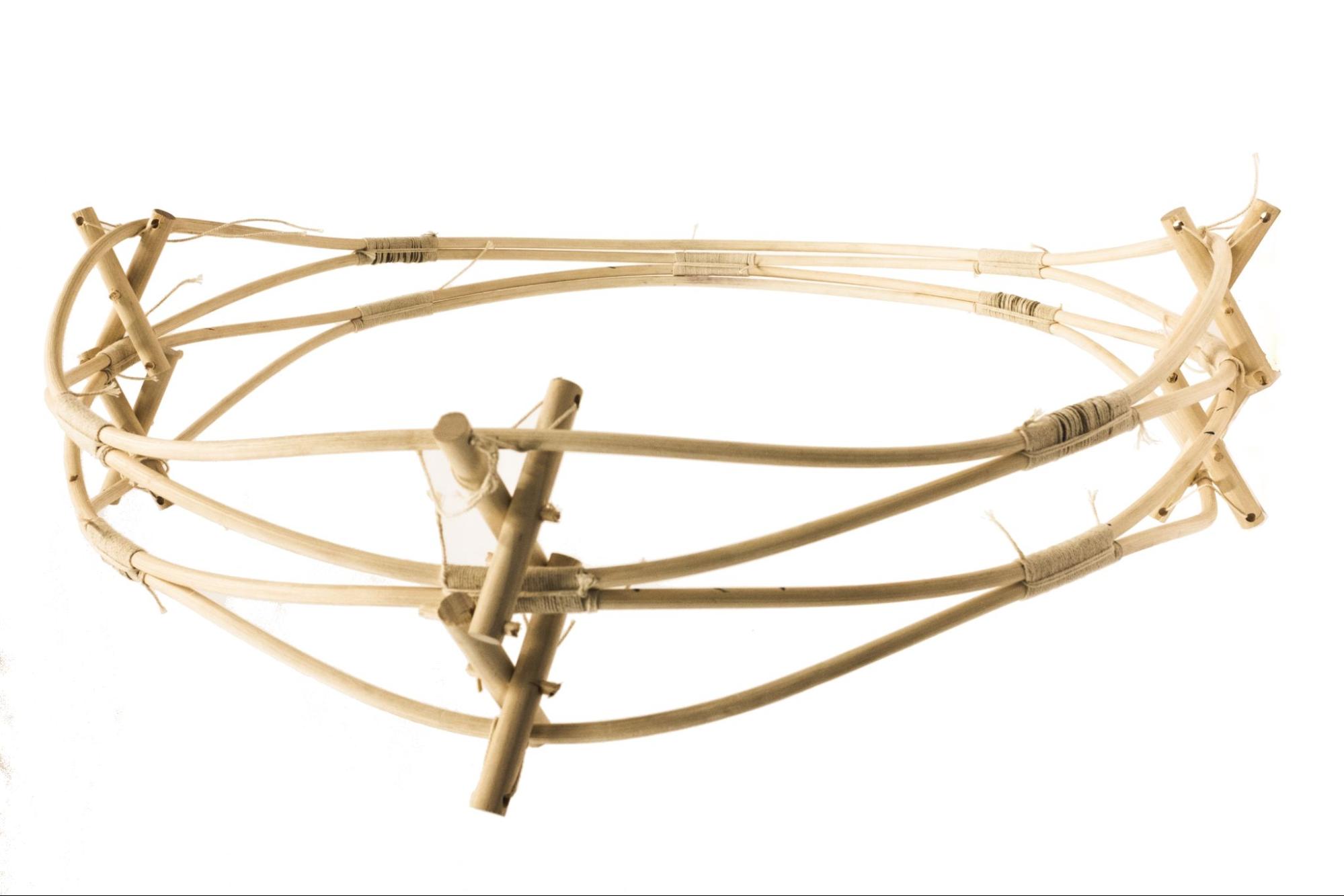
BAMBOO X-POD: PHASE I
Bamboo X-POD, an expandable structure for rapid deployment of an emergency shelter for climate refugees, is being prototyped using bamboo and bio-fibers. It deploys from a 2D to a 3D spatial structure using structural methods of deployment which include spacers, hinged arches, scissors-devices or cables. Hygroscopic forming, like wetting and drying, show promise. Scaled prototype studies used small diameter reeds with bio- fiber connections. Bamboo X-POD is a sequel to its metal precedent X-POD138 (2014) and part of the broader X-Bamboo expanded structure.











[Credits:]
Principal Investigator: Haresh Lalvani
UG Research Assistants: Tron Le, Guiben Zheng, Dillon Marlow, Tianyi Wang, Anna Kondrashova
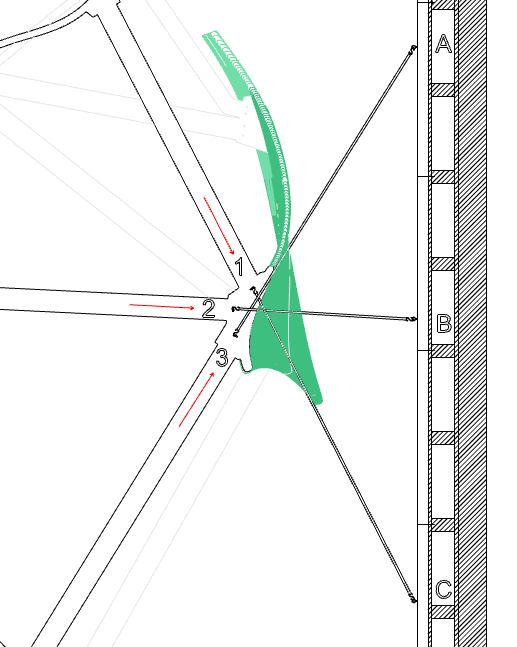
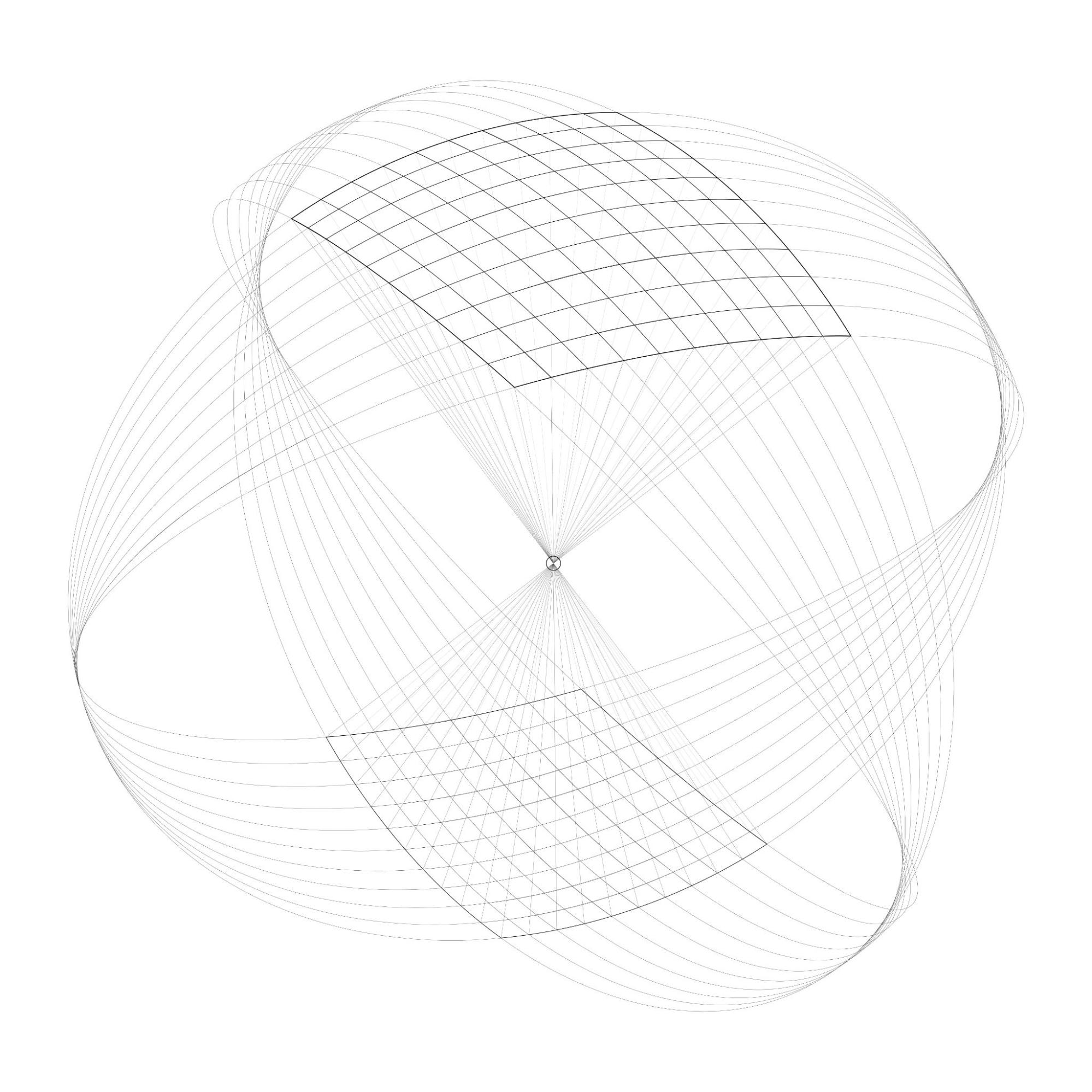
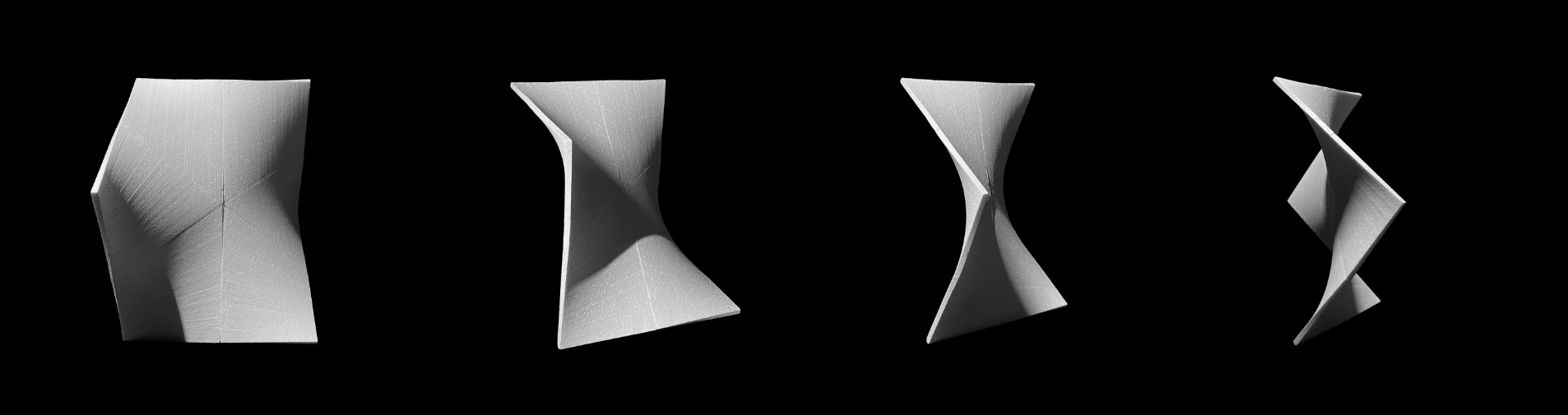
FRACTAL RULED SURFACES
We continue our explorations of 3D hyperstructures using robotically wire-cut modules to build periodic, non-periodic and asymmetric curved surfaces. We show two examples of space-filling surfaces (n=6 case) based on the pentagonal prism and the icosahedron using cells of the diamond lattice. Their 3D cells, saddle hex panels (p=6 case) and cell clusters are shown with thin wire-cut foam panels and its modules. All bounding surfaces of all modules are ruled surfaces. Precedents of ruled surfaces include the works of Gaudi, Candela and Calatrava.

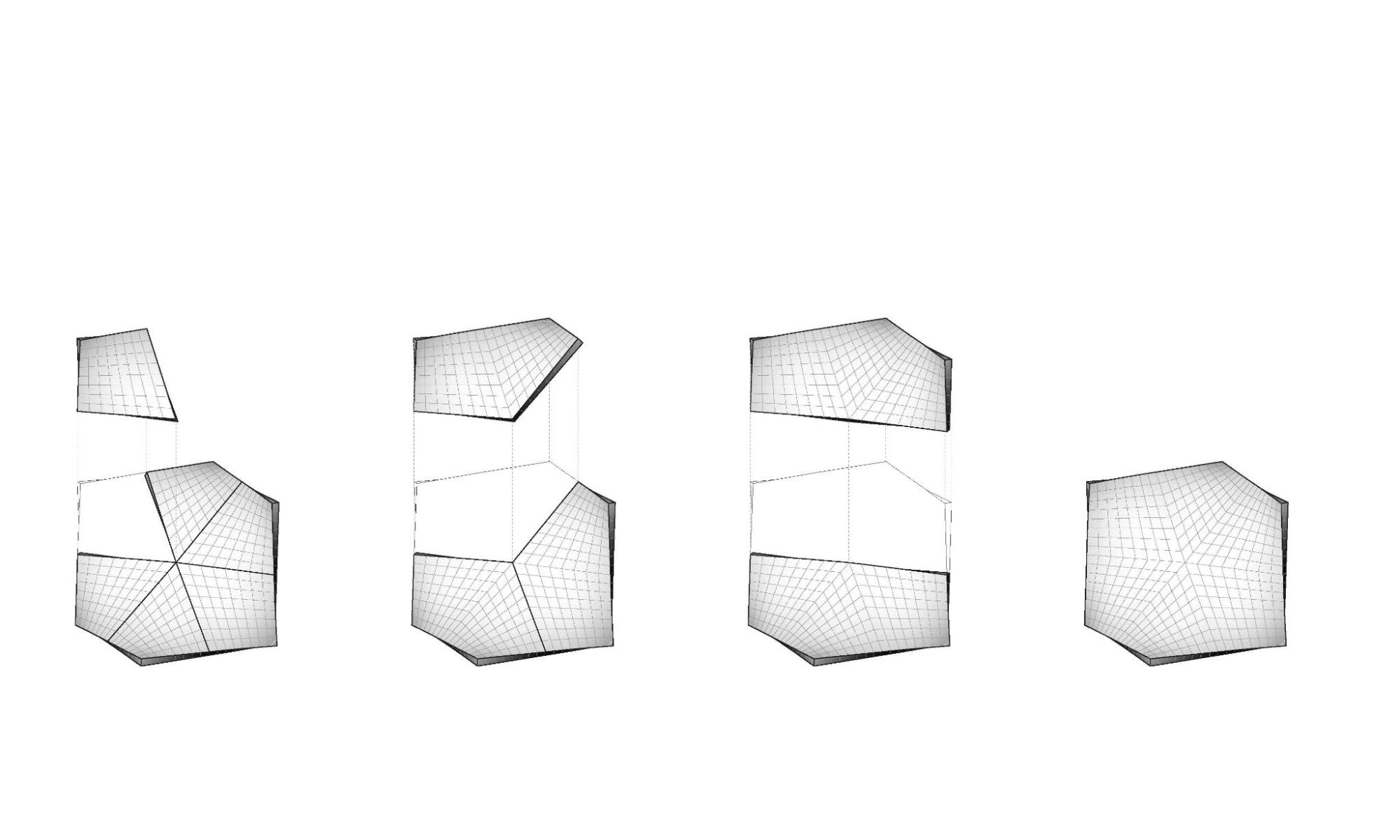
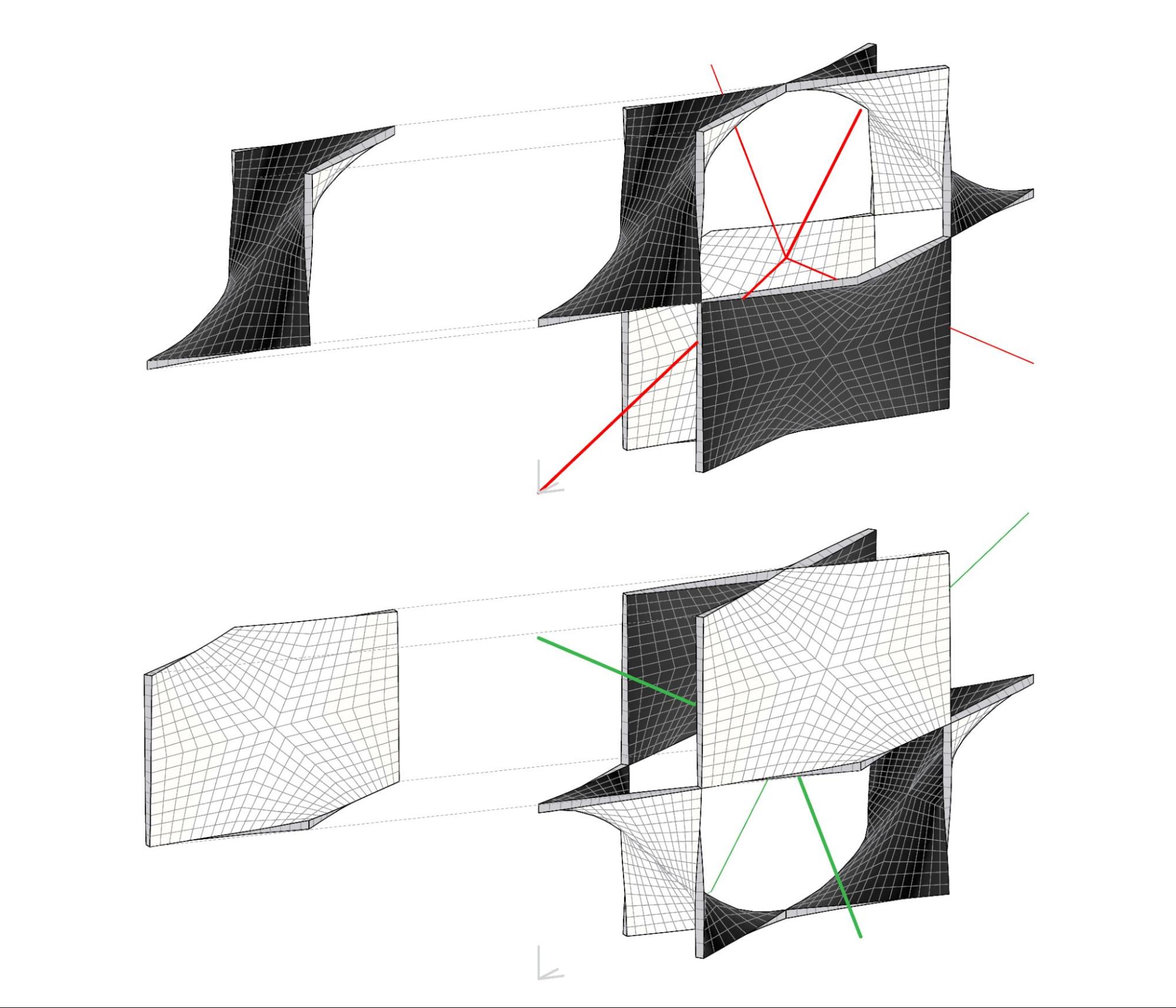
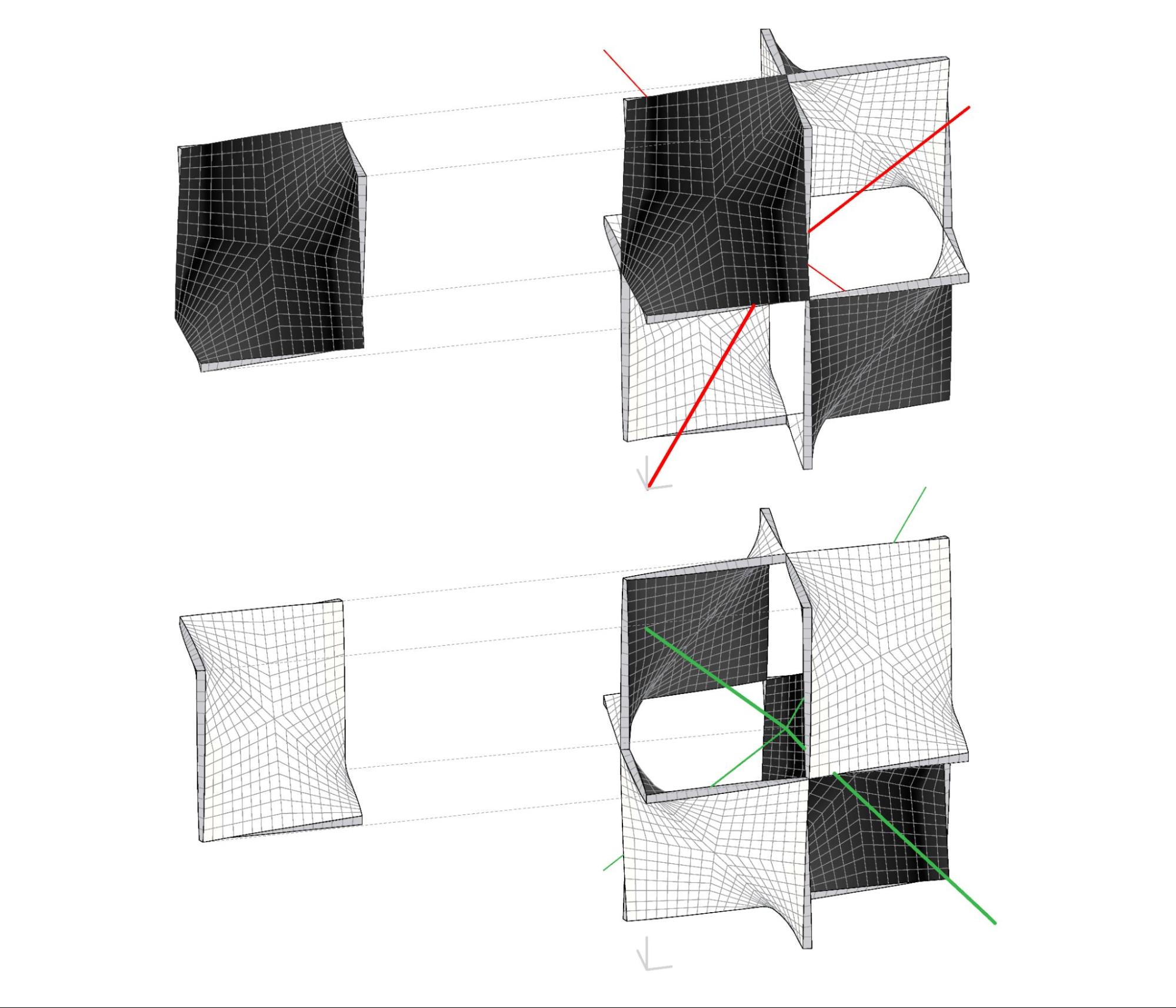
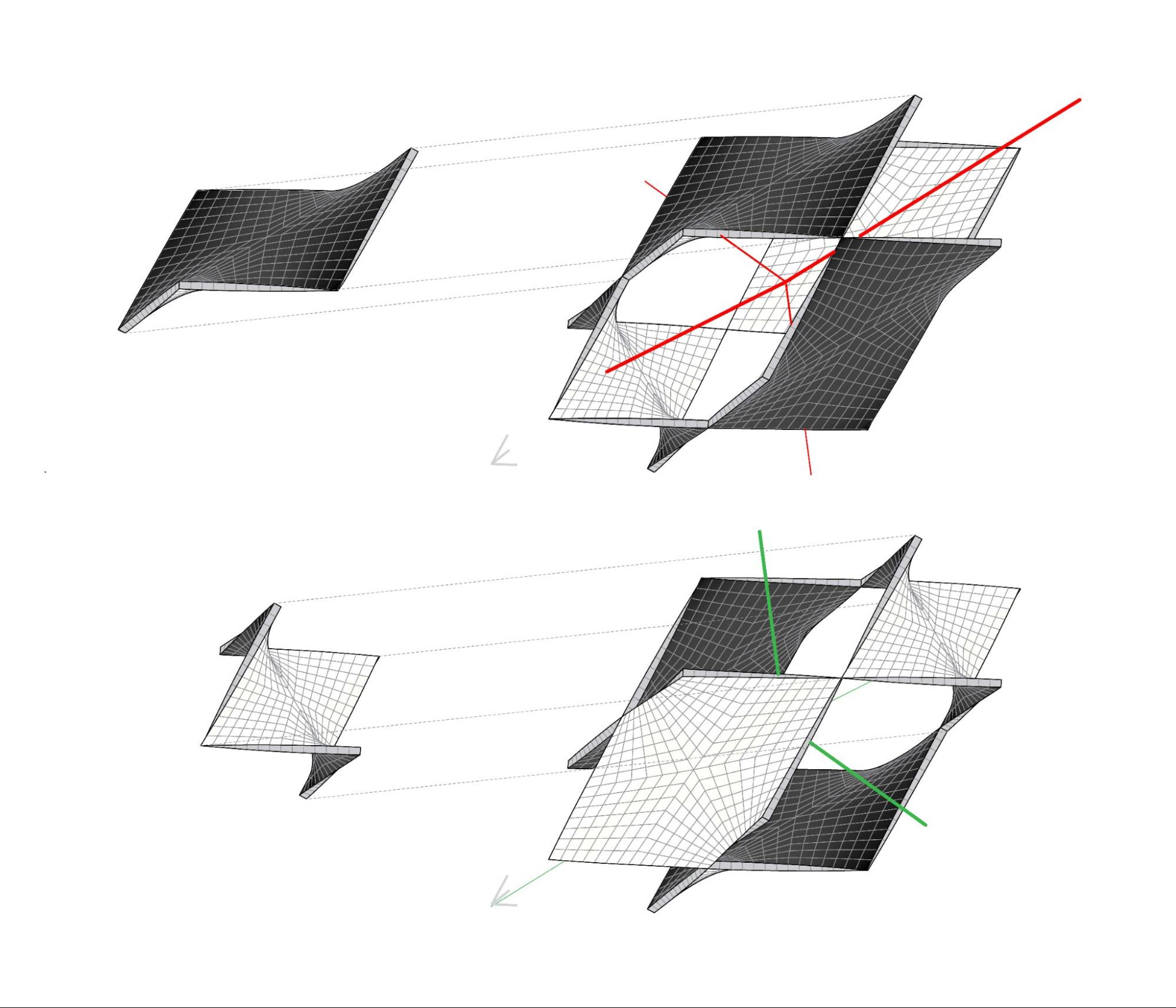
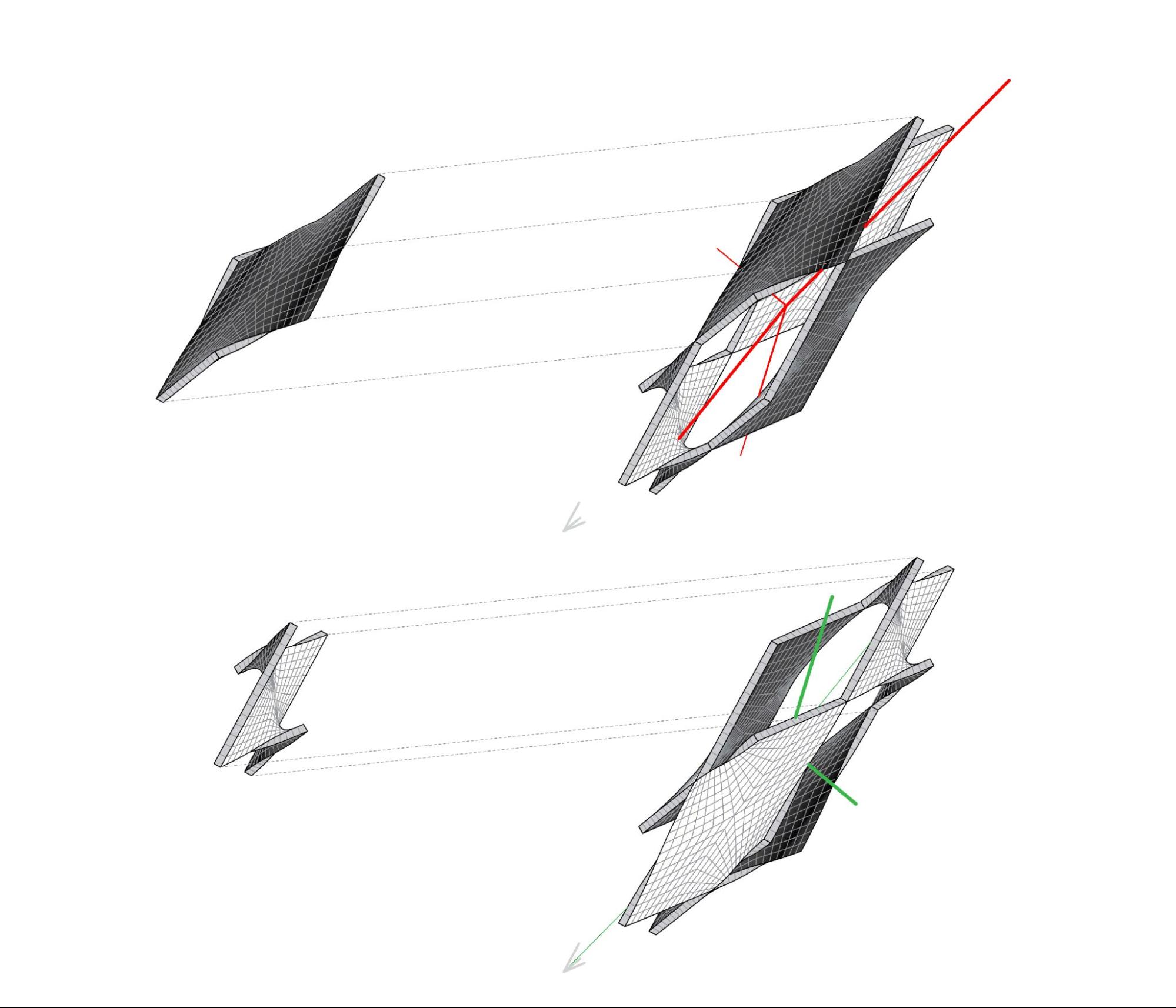
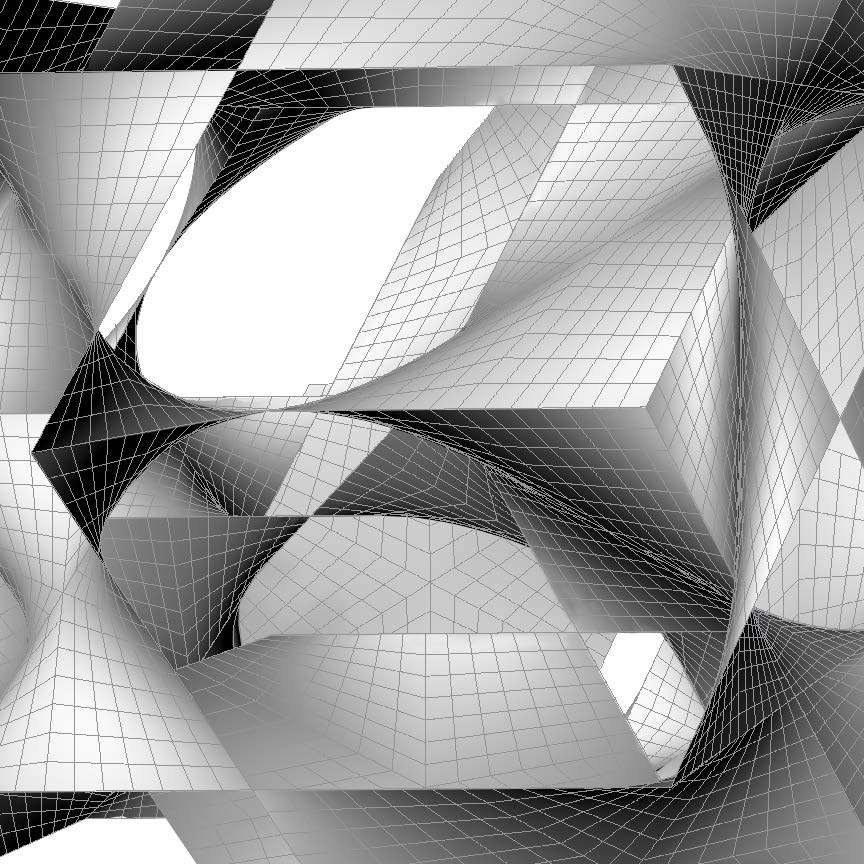
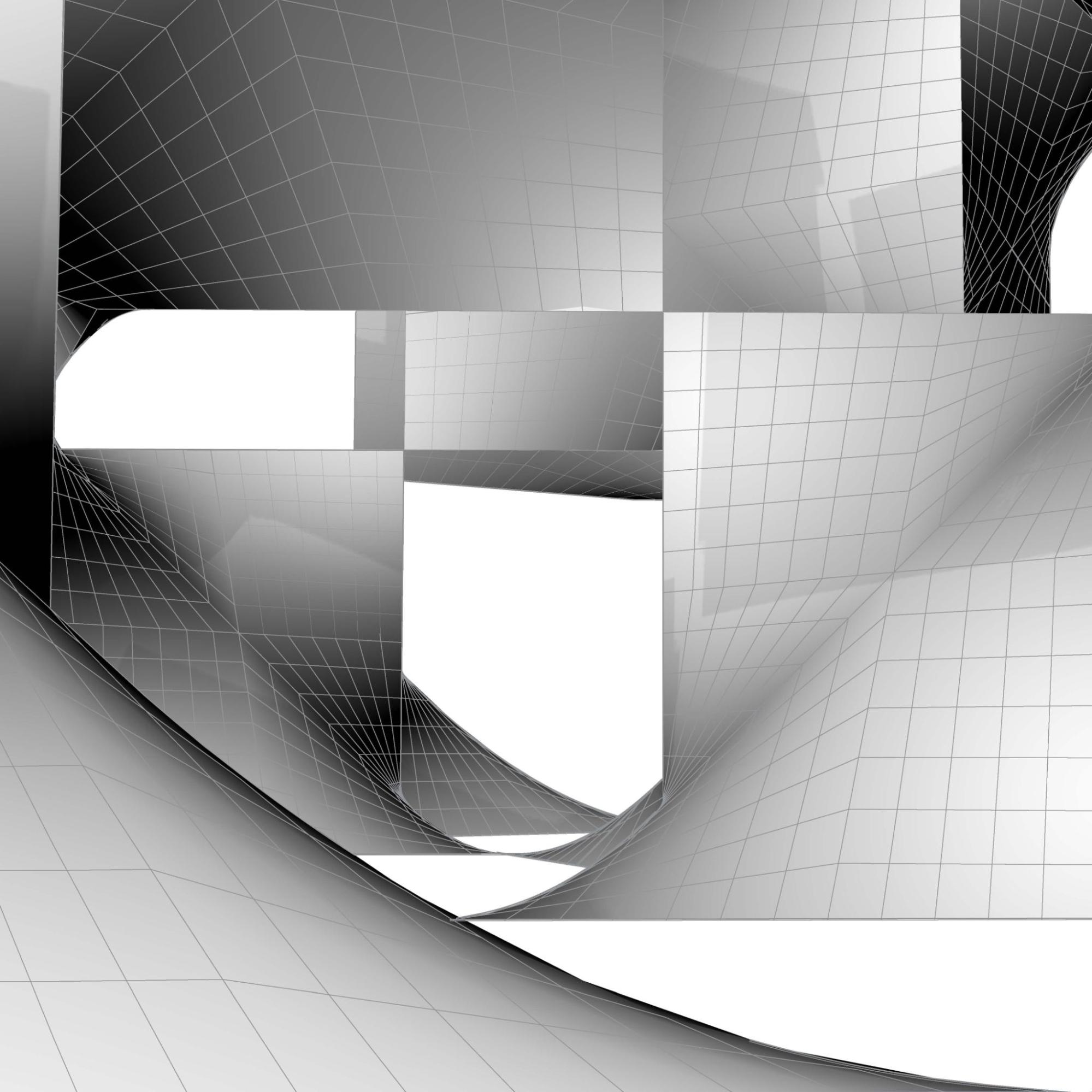
[Credits:]
Principal Investigator: Haresh Lalvani
Research Intern: Ahmad Tabbakh
UG Research Assistants: Ashkan Eslamifard, Jiayue “Chloe” Ni, Ahan Patil, Tyler Haas
